
If a point P (x, y) is rotated through 90° anti-clockwise about the origin to the point P', then co-ordinates of P' are (-y,x). It is common to label each corner with letters, and to use a little dash (called a Prime) to mark each corner of the reflected image.If a point P (x, y) is rotated through 90° clockwise about the origin to the point P', then co-ordinates of P' are (y,-x).

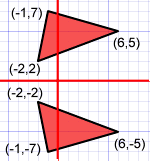
One can also think of the line or axis as a fold that perfectly folds the figure in half. If a point P (x, y) is rotated through 180° (clockwise or anti-clockwise) about the origin to the point P', then co-ordinates of P' are (-x,-y). The line of symmetry is the line or axis that is drawn on the figure to obtain symmetry.(iii) Reflection of P(x, y) in the origin is P'(-x, -y). (ii) Reflection of P(x, y) in the y-axis is P'(-x, y). Triangle DEF is formed by reflecting ABC across the x-axis and has vertices D (-6, -2), E (-4, -6) and F (-2, -4). KEYWORDS: Lesson Plans, Symmetry and Reflection Geometry Learning Units - High. Triangle ABC has vertices A (-6, 2), B (-4, 6), and C (-2, 4). of Square Root Functions (Reflection in the x-axis): Determining the. (i) Reflection of P(x, y) in the x-axis is P'(x, -y). x-axis reflection A reflection across the x-axis changes the position of the y-coordinate of all the points in a figure such that (x, y) becomes (x, -y). To find the reflection (or image) of a point P in a line ABįrom P, draw PM perpendicular to AB and produce PM to P' such that MP'= MP, then P' is the reflection (or image) of P in the line AB.The reflection (or image) of a point P in a given point M is a point P' such that M is the mid-point of the line segment PP'.The reflection (or image) of a point P in a line AB is a point P' such that AB is the perpendicular bisector of the line segment PP'.


 0 kommentar(er)
0 kommentar(er)
